next up previous contents
Next: Correlations in random number Up: Correlations in random numbers Previous: Correlations in random numbers   Contents

The distribution of this walues are shown in following pictures with comparision with p \( _{\chi ^{2},\nu }(w)\protect \) for \( \nu \protect \)=k(k-2)+1= 225 degrees of freedom.



\resizebox*{1.2\columnwidth}{!}{\includegraphics{pic/unragoodch.ps}}





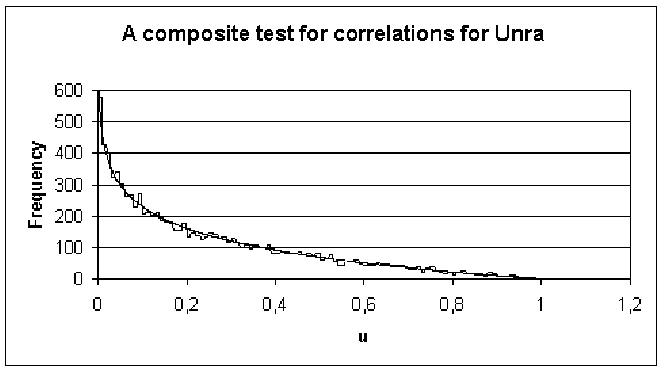
\resizebox*{1.2\columnwidth}{!}{\includegraphics{pic/rangoodch.ps}}



Super duper : normal and cumulative distributions



\resizebox*{1.3\columnwidth}{!}{\includegraphics{pic/supunifko.ps}}



We formed also the corresponding cumulative distributions and apply Kolmogorov-Smirnov test.



\resizebox*{1.3\columnwidth}{!}{\includegraphics{pic/unragoodko.ps}}





\resizebox*{1.3\columnwidth}{!}{\includegraphics{pic/rangoodk.ps}}



Kolmogorov-Smirnov statistics:

The f is probability of getting a value larger than q in a sample of size 200 in comparison of cumulative distributions.

next up previous contents
Next: Correlations in random number Up: Correlations in random numbers Previous: Correlations in random numbers   Contents
Amaury LATAILLADE 2002-11-04